Fiber Simulation Software

Of the many modes of fibers, the guided modes are of particular interest, as they are important e.g. for coupling light into a fiber and for the beam quality of the output. The mode properties are determined by the refractive index profile of the fiber. Properties of interest can be, for example,

• the shapes of the amplitude or intensity distribution of modes
• mode radii and effective mode areas
• the overlap with the doped core in a rare-earth-doped fiber
• effective refractive indices
• chromatic dispersion, e.g. the group velocity dispersion versus wavelength (calculated by differentiation of the frequency-dependent phase delay)
• bend losses versus bend radius

Most all-glass fibers have a radially symmetric refractive index profile and also exhibit only weak index contrasts, so that approximations for weakly guiding waveguides can be used. Under these circumstances, the LP modes of the fiber can be calculated with a mode solver based on relatively simple numerical methods. This also means that the calculation can be rather fast – the complete guided mode properties can be calculated within a second on an ordinary PC, even if there are many such modes.

Figure 1 shows an example for a few-mode fiber, where the wavelength is varied. While for the shortest wavelength (750 nm) the fiber has modes with 6 different intensity profiles, more and more modes disappear for longer wavelengths, until the fiber is single-mode.

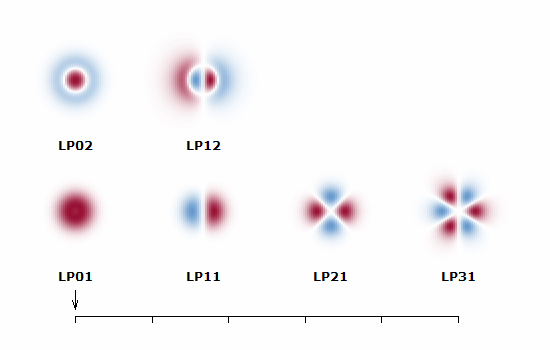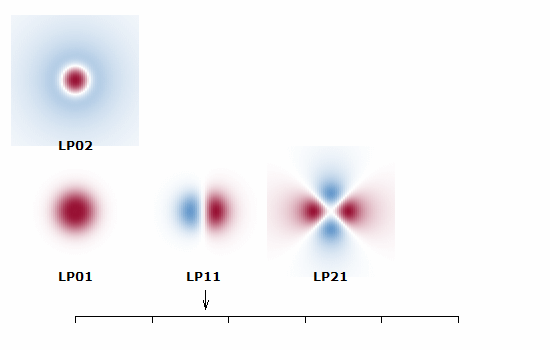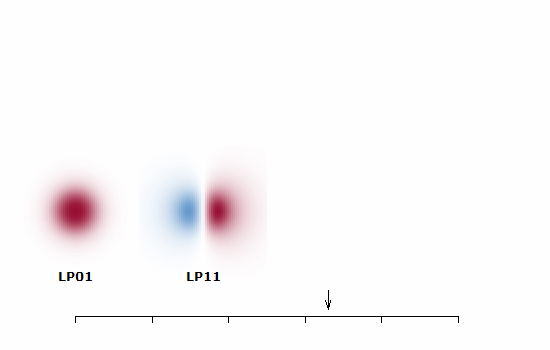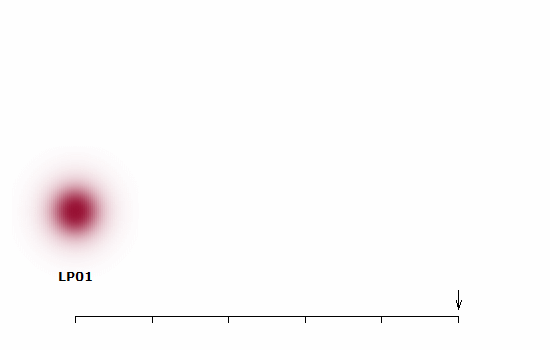
Mode solvers based on substantially more sophisticated algorithms are required for other cases, such as polarization-maintaining fibers lacking radial symmetry, and in particular for photonic crystal fibers. The latter also exhibit very strong index contrasts, i.e., the weakly guiding approximation can not be applied. There are various numerical techniques, and generally the computation time is much longer than for the simple methods as normally used for all-glass fibers.

Not in all situations, the calculation of fiber modes is both feasible and helpful. For example, the mode calculation is very difficult and rather time-consuming for the pump claddings of some double-clad fibers with irregular profile. It also gives more detailed information than required (hundreds or thousands of detailed mode intensity profiles), while still not fully taking into account the effects of irregular fiber bends. Another example is the behavior of fused fiber couplers, where the modes strongly depend on the local conditions. In such cases, it is often more suitable to numerical simulate the beam propagation.

As a first example, Figure 2 shows how pump light gets absorbed in a double-clad fiber with a simple geometry: a circular core in the middle of a circular pump cladding. Here, pump absorption is quite incomplete as some modes have very poor overlap with the doped core. Such a beam propagation model can be easily applied to other geometries, e.g. with an off-centered core, a D-shaped or octagonal core, or with strong bending, which may even be variable along the fiber length.

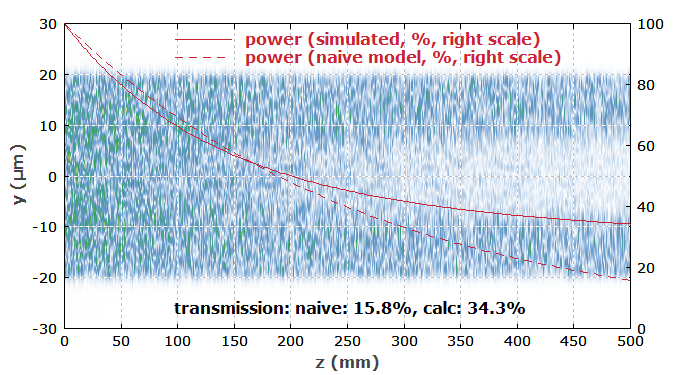
Another example is a fused fiber coupler, where two cores come close together over some distance. Their evanescent fields then touch each other, so that light can couple over. The detailed behavior depends strongly on the gap size, the coupling distance, and the wavelength. Figure 3 shows a typical simulation.

Optical amplification occurs in fiber lasers and amplifiers, which are usually based on rare-earth-doped fibers. The dopant is usually restricted to the fiber core, but there are also modified designs for special applications e.g. with ring doping around the core.

Behavior of the Laser-active Ions

Laser or Amplifier Gain

Self-consistency in Fiber Lasers and Amplifiers

In various cases, not the steady state is of interest, but rather the temporal evolution for time-dependent power inputs. As an example, one may consider a fiber amplifier which is first pumped for some time, so that some energy is stored in the fiber, and then serves to amplify an injected nanosecond pulse. During the pumping phase, the gain raises while the pump absorption decreases; the transmitted residual pump power may increase with time. When the signal pulse is injected, it will saturate the gain, so that the temporal shape of the output pulse can become quite distorted.

Figure 5 shows an example, where a signal input pulse with super-Gaussian temporal shape extracts much of the energy stored in an ytterbium-doped amplifier. The dot-dashed curve shows how the output would evolve without gain saturation. Due to gain saturation, however, the gain drops during the amplification, and the output power gets lower.

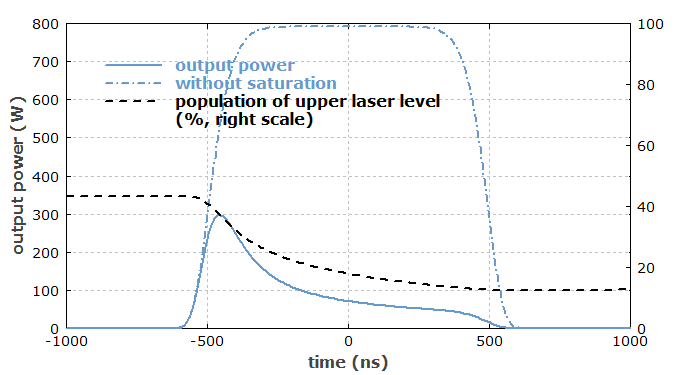
Another example is a Q-switched fiber laser. Here, it is essential to take into account propagation times (which can often be neglected in amplifier models). Lasing begins with ASE, which can be spatially quite variable due to the high gain which fiber devices typically have. As a result, the generated output can exhibit complicated peak structures, which are not observed for Q-switched bulk lasers. Figure 6 shows an example case, where a very fast Q-switch has been assumed. For slower switching, one can obtain quite different pulse shapes.

Note that rather different time scales can be involved in a simulation. Pumping can usually be simulated with rather course numerical time steps, whereas the generation of a Q-switched pulse requires much finer steps. A software which can work only with a fixed step size would then be extremely time-consuming.

Ultrashort pulses can be amplified in a fiber amplifier or generated in a mode-locked fiber laser. Also, one may want to investigate pulse propagation in passive fibers, as used e.g. for delivery to an application. For pulse propagation in the fiber, additional effects need to be taken into account – in particular, the chromatic dispersion and the fiber's nonlinearities (possibly including stimulated Raman scattering). In addition, it may be necessary to treat multiple fibers with different properties in a model and also effects of additional components, such as a saturable absorber for mode locking, an optical filter or an output coupler. (With such features, a fiber simulation software also becomes usable for many bulk-optical devices.)

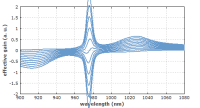
As an example, Figure 7 shows how the optical spectrum of the output pulse of a similariton-type mode-locked fiber laser converges to a nearly rectangular shape within 100 resonator round-trips. One also obtains the spectral phase, which is close to parabolic in the relevant region, so that dispersive pulse compression is not difficult to do.

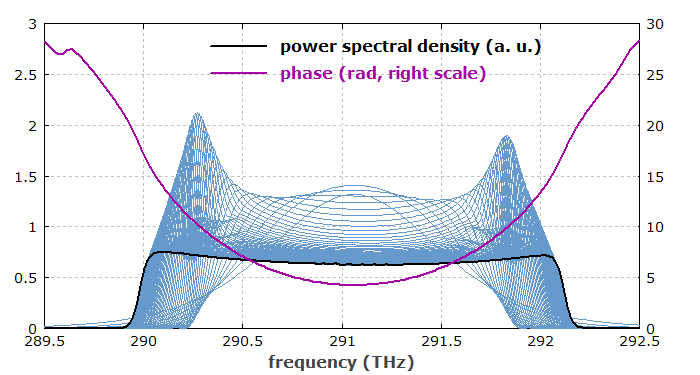
Generally, ultrashort pulse propagation is substantially more time-consuming to simulate, compared with simple propagation of powers for continuous-wave cases. For finding the steady state e.g. for the amplification of high-repetition rate pulse trains, it is therefore much more efficient to first do a steady-state calculation and then refine the solution as required with ultrashort pulse propagation.

As the resulting processes can be rather complex, it is very desirable to have flexible tools both for arranging different model situations and for inspecting the results. For example, it is useful to inspect the pulses at any location and after any number of round trips in the time and frequency domain, and to store pulses at specified locations for later analysis.

Your question or comment:

Spam check:

  (Please enter the sum of thirteen and three in the form of digits!)

By submitting the information, you give your consent to the potential publication of your inputs on our website according to our rules. (If you later retract your consent, we will delete those inputs.) As your inputs are first reviewed by the author, they may be published with some delay.