Spectral Phase
Definition: the phase of the electric field in the frequency domain
German: spektrale Phase
Categories: light detection and characterization, optical metrology, light pulses
Formula symbol: φ
Units: rad
How to cite the article; suggest additional literature
Author: Dr. Rüdiger Paschotta
The electric field of an optical pulse may be described in the time domain or in the frequency domain. In the frequency domain, it can be of interest to know not only the power spectral density (i.e., the intensity spectrum) but also the spectral phase. This is defined as the phase of the electric field in the frequency domain, i.e., the complex phase of the function
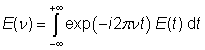
Complete pulse characterization includes measuring not only the optical spectrum, i.e. the squared modulus of E(ν), but also the spectral phase, which contains additional information. This is possible e.g. with the methods of frequency-resolved optical gating (FROG) and spectral phase interferometry for direct electric-field reconstruction (SPIDER, → spectral phase interferometry).
Spectral Phase and Group Delay
The group delay can be defined as the derivative of the spectral phase with respect to angular frequency. This means e.g. that the group delay of all spectral components is zero if the spectral phase is constant. If this is not the case, the group delay may be frequency dependent: different frequency components can then be considered to arrive at different times. However, this kind of interpretation is somewhat problematic, as becomes apparent e.g. when considering that the instantaneous frequency may acquire a certain value more than once within the pulse duration, whereas the group delay for a particular frequency can have only one value. For simple pulse shapes, however, the group delay can be used to describe the position of the pulse maximum of the whole pulse, or of the pulse obtained after a bandpass filter.
Examples
It is instructive to consider the changes of spectral phase associated with certain operations:
- A constant change in temporal phase translates directly into the same change in the spectral phase (for time-dependent phase changes, the relation is much less obvious).
- A time delay by T corresponds to a change in spectral phase which is 2π ν T, i.e. proportional to the optical frequency. In cases where the temporal position is not of interest, the spectral phase can be made less arbitrary by temporally shifting the pulse so that the linear term in frequency vanishes.
- Chromatic dispersion directly affects the spectral phase. For example, the effect of third-order dispersion corresponds to adding a term to the spectral phase which varies with the third power of the frequency offset.
When the spectral phase is constant or depends linearly on the frequency, the pulse is unchirped, which implies that it is at the transform limit. A chirp in the time domain is associated with a nonlinear frequency dependence of the spectral phase. A dispersive pulse compressor basically has the task of applying spectral phase shifts so that the resulting spectral phase is constant (or changes only linearly with frequency). The deviations from a flat spectral phase are more informative measure of the quality of pulse compression than e.g. just the pulse duration achieved.
The spectral phase can be useful for understanding the phenomenon of spectral interference. For example, consider two identical pulses with a relative time delay T. The difference in spectral phase, which is linear in frequency (see above), causes a spectral modulation. See the article on spectral phase interferometry for more details.
Modifying the Spectral Phase
There are pulse shapers which can be used to modify the spectral phase of pulses. Such a setup consists of, e.g., a first diffraction grating to separate different frequency components spatially, a liquid crystal modulator for applying position-dependent phase shifts, and a second diffraction grating to recombine the frequency components.
By properly adjusting all the phase values, it is possible e.g. to obtain transform-limited pulses, being as short as the given spectral width allows, or to form longer pulses with complicated temporal shapes. Conditions for such capabilities are that the full optical bandwidth can be processed, and that the spectral resolution (related to the maximum occurring group delay) is sufficiently high. On the other hand, a fast optical modulator is not required.
Questions and Comments from Users
Here you can submit questions and comments. As far as they get accepted by the author, they will appear above this paragraph together with the author’s answer. The author will decide on acceptance based on certain criteria. Essentially, the issue must be of sufficiently broad interest.
Please do not enter personal data here; we would otherwise delete it soon. (See also our privacy declaration.) If you wish to receive personal feedback or consultancy from the author, please contact him e.g. via e-mail.
By submitting the information, you give your consent to the potential publication of your inputs on our website according to our rules. (If you later retract your consent, we will delete those inputs.) As your inputs are first reviewed by the author, they may be published with some delay.
Bibliography
| [1] | J. P. Heritage et al., “Picosecond pulse shaping by spectral phase and amplitude manipulation”, Opt. Lett. 10 (12), 609 (1985), doi:10.1364/OL.10.000609 |
| [2] | I. A. Walmsley and V. Wong, “Characterization of the electric field of ultrashort optical pulses”, J. Opt. Soc. Am. B 13 (11), 2453 (1996), doi:10.1364/JOSAB.13.002453 |
| [3] | C. Iaconis and I. A. Walmsley, “Spectral phase interferometry for direct electric-field reconstruction of ultrashort optical pulses”, Opt. Lett. 23 (10), 792 (1998), doi:10.1364/OL.23.000792 |
See also: optical phase, chirp, transform limit, pulse characterization, spectral phase interferometry, pulse compression, double pulses
and other articles in the categories light detection and characterization, optical metrology, light pulses
 |
If you like this page, please share the link with your friends and colleagues, e.g. via social media:
These sharing buttons are implemented in a privacy-friendly way!