Frequency-resolved Optical Gating
Acronym: FROG
Definition: a technique for the complete characterization of ultrashort optical pulses
Categories: light detection and characterization, optical metrology, light pulses, methods
How to cite the article; suggest additional literature
Author: Dr. Rüdiger Paschotta
Frequency-resolved optical gating [1, 3] is a technique for the “complete” characterization of ultrashort pulses, i.e. for measuring not only pulse parameters such as the pulse energy or pulse duration, but also the full time-dependent electric field or (equivalently) the optical spectrum including the frequency-dependent spectral phase. (The carrier–envelope offset and the arrival time of pulses can not be measured.) This technique has been pioneered by Rick Trebino's research group at the Georgia Institute of Technology.
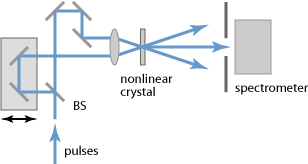
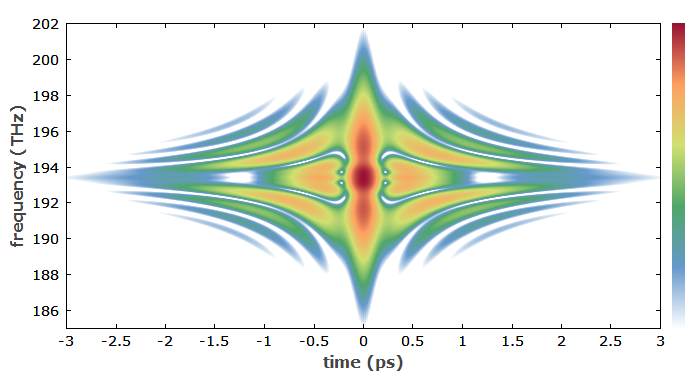
A typical setup for a FROG measurement (Figure 1) is similar to that of an intensity autocorrelator, except that the photodetector is replaced with a spectrograph. A FROG measurement involves recording some tens or hundreds of spectra for different settings of the arrival time difference of the two pulses. These data can be illustrated in the form of a so-called FROG trace (see Figure 2), which is a kind of spectrogram and displays with a color scale the intensity as a function of time delay and optical frequency (or wavelength).
A sophisticated iterative phase retrieval algorithm, implemented as a computer program, can then be used for reconstructing the pulse shape from the FROG trace. As the recorded data are substantially redundant, the FROG retrieval algorithm can not only deliver the pulse shape, but also carry out a consistency check. It may thus be noticed when errors occur, e.g. due to wrong calibration of the spectrometer. On a typical PC, the procedure may take a few minutes with a non-optimized algorithm, and optimized algorithms have been developed which make it possible to do the calculations in less than 0.1 s, at least for simply shaped pulses.
The term “frequency-resolved optical gating” originates from the idea that a short gate pulse can be used to obtain a sample from a longer pulse by nonlinear mixing (gating) in a nonlinear crystal material. As an additional gate pulse, shorter than the pulse to be investigated, is usually not available, FROG actually uses the pulse itself for gating. This makes the method much simpler to apply, but at the same time conceptually and computationally more sophisticated.
Variants of Frequency-resolved Optical Gating
There are different versions of FROG, which rely on different nonlinear gating mechanisms, generate different kinds of FROG traces (thus requiring different phase retrieval algorithms), and have different strengths and weaknesses:
- Polarization-gated FROG (PG FROG) is the conceptually simplest FROG variant. Here, the gate pulse, polarized under 45° relative to the probe pulse, rotates the polarization of the latter when overlapping it in a χ(3) medium (e.g. fused silica), and thus leads to transmission of the probe through a polarizer. As always with FROG, the transmitted probe signal is analyzed with a spectrometer. Advantages of PG FROG are easy alignment, the absence of ambiguities in the retrieval, and the generation of fairly intuitive FROG traces. A problem is that a polarizer with very high extinction ratio is required.
- In self-diffraction FROG (SD FROG), two beams overlapping in a χ(3) medium generate a nonlinear refractive index grating, which diffracts both beams into new beams, one of which is used for detection. As no polarizers are required, SD FROG can be applied in various spectral regions, e.g. in the deep UV region. However, relatively high pulse energies are required.
- Transient-grating FROG (TG FROG) also uses a nonlinear refractive index grating, but uses a third pulse with variable delay as the probe which is diffracted at the grating generated by the other two beams. For various reasons, it allows for a much higher detection sensitivity than SD FROG.
- Second-harmonic FROG (SHG FROG) is the most popular FROG variant. It is based on a χ(2) nonlinear crystal and can thus reach a much higher sensitivity than is possible with all χ(3) versions of FROG. Phase-matching issues have to be carefully treated to avoid distortions for short pulses. There is an ambiguity concerning the direction of time, which can be removed e.g. by performing an additional measurement with some glass piece in the beam path.
- Interferometric FROG (IFROG) [12] uses a collinear geometry, avoiding a loss of temporal resolution via geometric effects (finite beam angles) in the characterization of few-cycle pulses.
- Cross-correlation FROG (XFROG) [7] uses an additional reference pulse, which does not need to be spectrally overlapping with the pulse under investigation. The recorded signal is obtained by sum or difference frequency generation of the two pulses. This method can be very sensitive, and can be applied in different spectral regions.
Beyond these traditional FROG measurement methods, refined versions of FROG have been developed, which can be applied even to very short pulses (with angle dithering of the crystal to remove strong effects of group velocity mismatch in the nonlinear crystal) or to fairly long pulses (where a high spectrometer resolution is required). A particularly compact setup is achieved with the GRENOUILLE geometry [9], which has no moving parts and even allows the measurement of additional features such as spatial chirps. A waveguide as the nonlinear component allows detection at ultralow power levels, and polarization scrambling makes possible polarization-independent measurements, which facilitate e.g. the delivery of pulses via fibers [13].
A possible alternative to frequency-resolved optical gating is spectral phase interferometry for direct electric-field reconstruction (SPIDER), as explained in the article on spectral phase interferometry.
Suppliers
The RP Photonics Buyer's Guide contains 11 suppliers for frequency-resolved optical gating devices. Among them:


APE
Second Harmonic Generation FROG is the most popular spectrometer-less Frequency-Resolved Optical Gating method. Most of the pulseCheck autocorrelators by APE optionally integrate FROG and give access to complete pulse characterization. The addition of a special nonlinear crystal module and dedicated software opens the door to complete spectral and temporal pulse characterization. The FROG Option enables the pulseCheck to measure the spectral and temporal bandwidth and phase with just a few adjustments to the autocorrelator.
The FROG Option consists of FROG crystal(s), a plane FROG mirror and FROG measurement and retrieval software.


Swamp Optics
Frequency-Resolved Optical Gating (FROG) is the gold standard of laser pulse measurement. It yields the complete intensity and phase vs. time for an arbitrary pulse. GRENOUILLE is a simple, elegant version of FROG.
Swamp Optics sells commonly used configurations from stock. It also provides customs solutions for more exotic wavelength and pulse durations.
Questions and Comments from Users
Here you can submit questions and comments. As far as they get accepted by the author, they will appear above this paragraph together with the author’s answer. The author will decide on acceptance based on certain criteria. Essentially, the issue must be of sufficiently broad interest.
Please do not enter personal data here; we would otherwise delete it soon. (See also our privacy declaration.) If you wish to receive personal feedback or consultancy from the author, please contact him e.g. via e-mail.
By submitting the information, you give your consent to the potential publication of your inputs on our website according to our rules. (If you later retract your consent, we will delete those inputs.) As your inputs are first reviewed by the author, they may be published with some delay.
Bibliography
| [1] | D. J. Kane and R. Trebino, “Characterization of arbitrary femtosecond pulses using frequency-resolved optical gating”, IEEE J. Quantum Electron. 29 (2), 571 (1993), doi:10.1109/3.199311 |
| [2] | D. J. Kane and R. Trebino, “Single-shot measurement of the intensity and phase of an arbitrary ultrashort pulse by using frequency-resolved optical gating”, Opt. Lett. 18 (10), 823 (1993), doi:10.1364/OL.18.000823 |
| [3] | FROG tutorial of Rick Trebino's group at the Georgia Institute of Technology, http://www.frog.gatech.edu/tutorial.html |
| [4] | K. W. DeLong et al., “Frequency-resolved optical gating using second-harmonic generation”, J. Opt. Soc. Am. B 11 (11), 2206 (1994), doi:10.1364/JOSAB.11.002206 |
| [5] | R. Trebino et al., “Measuring ultrashort laser pulses in the time–frequency domain using frequency-resolved optical gating”, Rev. Sci. Instrum. 68, 3277 (1997), doi:10.1063/1.1148286 |
| [6] | A. Baltuška et al., “Amplitude and phase characterization of 4.5-fs pulses by frequency-resolved optical gating”, Opt. Lett. 23 (18), 1474 (1998), doi:10.1364/OL.23.001474 |
| [7] | S. Linden et al., “Amplitude and phase characterization of weak blue ultrashort pulses by downconversion”, Opt. Lett. 24 (8), 569 (1999), doi:10.1364/OL.24.000569 |
| [8] | L. Gallmann et al., “Collinear type II second-harmonic-generation frequency-resolved optical gating for the characterization of sub-10-fs optical pulses”, Opt. Lett. 25 (4), 269 (2000), doi:10.1364/OL.25.000269 |
| [9] | P. O'Shea et al., “Highly simplified device for ultrashort-pulse measurement”, Opt. Lett. 26 (12), 932 (2001), doi:10.1364/OL.26.000932 |
| [10] | J. Zhang et al., “Measurement of the intensity and phase of attojoule femtosecond light pulses using Optical-Parametric-Amplification Cross-Correlation Frequency-Resolved Optical Gating”, Opt. Express 11 (6), 601 (2003), doi:10.1364/OE.11.000601 |
| [11] | S. Akturk et al., “Extremely simple device for measuring 20-fs pulses”, Opt. Lett. 29 (9), 1025 (2004), doi:10.1364/OL.29.001025 |
| [12] | G. Stibenz and G. Steinmeyer, “Interferometric frequency-resolved optical gating”, Opt. Express 13 (7), 2617 (2005), doi:10.1364/OPEX.13.002617 |
| [13] | H. Miao et al., “Polarization-insensitive ultralow-power second-harmonic generation frequency-resolved optical gating”, Opt. Lett. 32 (7), 874 (2007), doi:10.1364/OL.32.000874 |
| [14] | X. Liu et al., “Numerical simulations of ultrasimple ultrashort laser-pulse measurement”, Opt. Express 15 (8), 4585 (2007), doi:10.1364/OE.15.004585 |
| [15] | D. Lee et al., “Experimentally simple, extremely broadband transient-grating frequency-resolved-optical gating arrangement”, Opt. Express 15 (2), 760 (2007), doi:10.1364/OE.15.000760 |
| [16] | J. Gagnon et al., “The accurate FROG characterization of attosecond pulses from streaking measurements”, Appl. Phys. B 92, 25 (2008), doi:10.1007/s00340-008-3063-x |
| [17] | R. Trebino, Frequency-Resolved Optical Gating: the Measurement of Ultrashort Laser Pulses, Kluwer, Boston (2002) |
See also: pulses, pulse characterization, autocorrelators, spectral phase interferometry
and other articles in the categories light detection and characterization, optical metrology, light pulses, methods
 |





If you like this page, please share the link with your friends and colleagues, e.g. via social media:
These sharing buttons are implemented in a privacy-friendly way!