Group Velocity Dispersion
Acronym: GVD
Definition: the frequency dependence of the group velocity in a medium, or (quantitatively) the derivative of the inverse group velocity with respect to angular frequency
More general term: chromatic dispersion
German: Gruppengeschwindigkeitsdispersion
Categories: general optics, fiber optics and waveguides, light pulses
Formula symbol: β2
Units: s2/m
How to cite the article; suggest additional literature
Author: Dr. Rüdiger Paschotta
Group velocity dispersion is the phenomenon that the group velocity of light in a transparent medium depends on the optical frequency or wavelength. The term can also be used as a precisely defined quantity, namely the derivative of the inverse group velocity with respect to the angular frequency (or sometimes the wavelength):
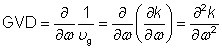
where k is the frequency-dependent wavenumber. (For waveguides, it is replaced with the phase constant β.)
The group velocity dispersion is the group delay dispersion per unit length. The basic units are s2/m. For example, the group velocity dispersion of silica is +35 fs2/mm at 800 nm and −26 fs2/mm at 1500 nm. Somewhere between these wavelengths (at about 1.3 μm), there is the zero-dispersion wavelength.
For optical fibers (e.g. in the context of optical fiber communications), the group velocity dispersion is usually defined as a derivative with respect to wavelength (rather than angular frequency). This can be calculated from the above-mentioned GVD parameter:
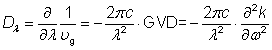
This quantity is usually specified with units of ps/(nm km) (picoseconds per nanometer wavelength change and kilometer propagation distance). For example, 20 ps/(nm km) at 1550 nm (a typical value for telecom fibers) corresponds to −25 509 fs2/m.
It is important to realize the different signs of GVD and Dλ, resulting from the fact that a long wavelength corresponds to a smaller optical frequency. In order to avoid confusion, the terms normal and anomalous dispersion can be used instead of positive and negative dispersion. Normal dispersion implies that the group velocity decreases for increasing optical frequency; this occurs in most cases.
Depending on the situation, group velocity dispersion can have different important effects:
- It is responsible for dispersive temporal broadening or compression of ultrashort pulses.
- In optical fibers, the effect of nonlinearities strongly depends on the group velocity dispersion. For example, there may be spectral broadening (even supercontinuum generation) or compression, depending on the dispersion properties.
- Dispersion is also responsible for the group velocity mismatch of different waves in parametric nonlinear interactions. For example, it can limit the interaction bandwidth in frequency doublers, optical parametric oscillators and amplifiers.
Questions and Comments from Users
2020-06-03
To calculate the GDD of a waveguide, would one just need to multiply GVD with the length of the waveguide?
Answer from the author:
Exactly.
Here you can submit questions and comments. As far as they get accepted by the author, they will appear above this paragraph together with the author’s answer. The author will decide on acceptance based on certain criteria. Essentially, the issue must be of sufficiently broad interest.
Please do not enter personal data here; we would otherwise delete it soon. (See also our privacy declaration.) If you wish to receive personal feedback or consultancy from the author, please contact him e.g. via e-mail.
By submitting the information, you give your consent to the potential publication of your inputs on our website according to our rules. (If you later retract your consent, we will delete those inputs.) As your inputs are first reviewed by the author, they may be published with some delay.
See also: chromatic dispersion, group delay dispersion, group velocity mismatch
and other articles in the categories general optics, fiber optics and waveguides, light pulses
 |
If you like this page, please share the link with your friends and colleagues, e.g. via social media: 
These sharing buttons are implemented in a privacy-friendly way! |
2020-05-12
I am wondering about the example numbers you are giving here. Specifically the −26 fs2/mm at 1500 nm; by using the calculator, I get 2.25 · 103 fs2/m. Why is there a difference or am I missing something here?
Answer from the author:
The calculator is not calculating the chromatic dispersion of silica, but only converting chromatic dispersion values given with different units. The value which you obtain just resulted from the conversion of the original value of −1.88 ps/(nm km) to the other units.