Passive Fiber Optics
This is part 3 of a tutorial on passive fiber optics from Dr. Paschotta. The tutorial has the following parts:
1: Guiding light in a glass fiber, 2: Fiber modes, 3: Single-mode fibers, 4: Multimode fibers, 5: Fiber ends, 6: Fiber joints, 7: Propagation losses, 8: Fiber couplers and splitters, 9: Polarization issues, 10: Chromatic dispersion of fibers, 11: Nonlinearities of fibers, 12: Ultrashort pulses and signals in fibers, 13: Accessories and tools
Part 3: Single-mode Fibers
In the previous part, we have seen that depending on its refractive index profile and the wavelength, a fiber may guide different numbers of modes. It may be only a single guided mode (the LP01 mode), if the numerical aperture and the refractive index contrast are small. In this regime, the fiber is called a single-mode fiber. Higher-order modes like LP11, LP20 etc. then do not exist – only cladding modes, which are not localized around the fiber core.
Note that in most cases light with different polarization states can be guided. The term “single-mode” ignores the fact that usually (for radially symmetric index profiles and no birefringence) one actually has two different modes with same intensity profile but orthogonal linear polarization directions. Any other polarization state can be considered as a linear superposition of these two. (See also part 6 on polarization issues.)
Condition for Single-mode Guidance
For step-index fiber designs, there is a simple criterion for single-mode guidance: the V number has to be below ≈2.405. The V number is defined as
where λ is the vacuum wavelength, a is the radius of the fiber core, and NA is the numerical aperture.
For other radial dependencies of the refractive index, or even for non radially symmetric index profiles, the single-mode condition normally has to be calculated numerically. It would not be correct to use the criterion V < 2.405, e.g. with V calculated from the maximum index difference.
Influence of the Core Size
Generally, one might expect that a smaller core implies a smaller fiber mode. For a constant V number, this is true; the index contrast is then getting smaller for a larger core. However, if we keep the numerical aperture constant, the V number changes with the core radius, and the mode radius shows a non-monotonic dependence on the core radius, as can be seen in Figure 2 for an NA of 0.1:
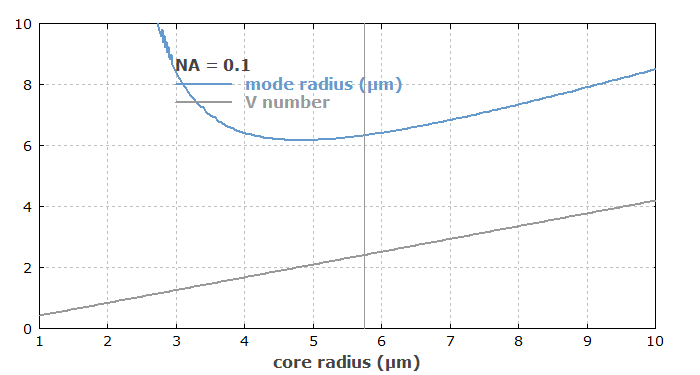
One can see that for core radii below ≈4.8 μm, where the V number gets rather small, the mode radius increases. In the regime of small V values, the mode extends far beyond the core, and it deviates substantially from a Gaussian shape.
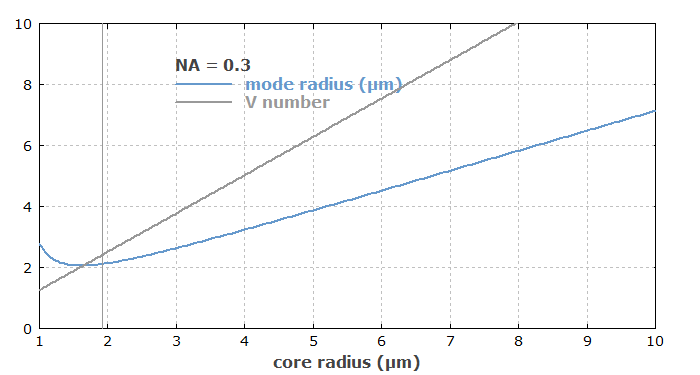
Figure 3 shows the same for a higher NA of 0.3:
Example: a Typical Single-mode Fiber
A typical kind of single-mode fiber for 1.5 μm wavelength may have a step-index profile with a core radius of 4 μm and a numerical aperture of 0.12. The guided mode then has a mode radius of 5.1 μm and an effective mode area of 75 μm2. That is not too far from the data of the often used SMF-28 telecom fiber from Corning.
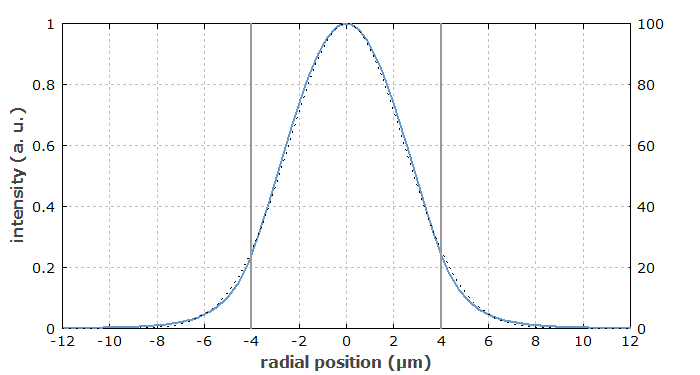
As is typical for single-mode fibers, the field distribution extends significantly beyond the core; only 54.4% of the power propagates in the core. (It may seem according to Figure 3 that it is more, but note the factor r in the area integral, which makes the outer parts of the profile more important.) However, the intensity drops quickly with increasing radial coordinate. The intensity profile is close to a Gaussian profile.
When we decrease the wavelength, we find that the fiber is no more single-mode below the cut-off wavelength of 1254 nm: in addition to the LP01 mode, it then also supports LP11 modes (actually two of these with orthogonal orientation). Below 787 nm, the LP02 mode comes in additionally.
In principle, the fiber stays single-mode for any wavelength above the LP11 cut-off, which is 1254 nm. However, for longer wavelengths the mode becomes larger and larger, and it will become increasingly sensitive to bend losses, resulting both from macroscopic bending and from microscopic imperfections. For the design discussed here, another problem is actually more serious: beyond ≈2 μm, absorption of the base material (silica) sets in. So in practice there is a limited wavelength interval into which a single-mode fiber can be used.
Launching Light into a Single-mode Fiber
Efficiently launching light into a single fiber modes requires that the complex amplitude profile of the incident light (assuming monochromatic light) has a high overlap with the corresponding mode amplitude profile. Fortunately, the fundamental mode of a single-mode fiber has in most cases a profile which is close to that of a Gaussian beam (for robust guiding, with large enough V value), and Gaussian beams are well approximated by the outputs of most single-mode lasers. So the remaining task is
- to properly focus a laser beam such that the beam radius is close to that of the fiber mode,
- to place the fiber end at the beam focus (beam waist), and
- to align the fiber such that the beam focus hits the fiber core with proper orientation.
Obviously, the position error of the incident beam should be small compared with the mode radius. The following formula tells how the launch efficiency (disregarding possible reflections of the interface) depends on the position error Δx and also on possible deviations between the input beam radius w1 and the mode radius w2, if we can assume Gaussian mode profiles:
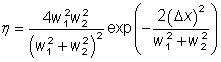
We see that for a perfect beam size, an offset of one beam radius reduces the coupling efficiency already to 1 / e ≈ 37%, and a 5 times smaller error allows for a 90% coupling efficiency. Note that the equation holds only for Gaussian profiles, but in most cases this is a good approximation.
The beam direction also has to be correct. This is not so sensitive, however, for typical single-mode fibers. The angle error should be well below the beam divergence, but that is relatively large for small more areas.
The Effect of Imperfect Launch Conditions
What happens, for example, if we somewhat misalign the input beam?
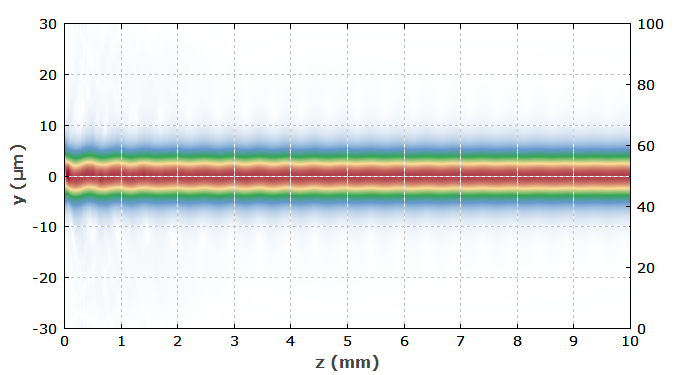
Figure 2 shows a simulated example, where the input laser beam is displaced by 1/10 of the beam radius. After some propagation length, only light in the guided mode remains. All other light is lost in the cladding. (The cladding/coating interface is often quite lossy.) At the end of a 10 cm long fiber, for example, one will find only light in the core, having a profile which is only determined by the mode profile. The launch conditions influence only the launched power, but not the output beam profile.
Obtaining Large Mode Areas
For some applications, it is desirable to have rather large mode areas while still having single-mode guidance. For example, one may want to minimize nonlinear effects or maximize the amount of stored energy in pulsed fiber amplifier while preserving a high beam quality.
In principle, it is easy to obtain single-mode guidance even for a rather large core: one only has to reduce the index contrast (thus the numerical aperture). For example, one could increase the core radius in the above example fivefold to 20 μm. If we reduce the numerical aperture by the same factor to 0.024, we still obtain single-mode guidance – now with an active area of 1869 μm2, which is 52 = 25 times larger than before. Unfortunately, however, that brings with it some trouble:
- As the index contrast is now extremely weak (0.0002), the fiber becomes extremely sensitive to small random index changes resulting from not perfectly controlled fabrication conditions. The NA of 0.024 is considered impractically small for currently available technology.
- Even if a perfect fiber is made based on that design, it will be very sensitive to bending. Figure 2 shows a numerical simulation, where the inverse bend radius grows linearly along the fiber. On the right side, a bend radius of 1 m is reached. Before that happens, the light experiences serious bend loss. This means that the fiber could be used only if it were essentially kept straight, and if any significant microbends could be avoided.

More advanced fiber designs have been developed, where mode areas well above 1000 μm2 can be obtained in combination with better bending properties and lower sensitivity to fabrication errors. However, the described problems cannot be fully avoided. The fundamental problem is that a very large mode is necessarily only weakly guided, and thus sensitive to various additional effects.
Efficient launching light into a large mode area single-mode fiber is simpler than for a small area, as far as the position alignment is concerned. Note, however, that as the beam divergence gets smaller, the angular alignment becomes more sensitive.
Go to Part 4: Multimode Fibers or back to the start page.
Questions and Comments from Users
Here you can submit questions and comments. As far as they get accepted by the author, they will appear above this paragraph together with the author’s answer. The author will decide on acceptance based on certain criteria. Essentially, the issue must be of sufficiently broad interest.
Please do not enter personal data here; we would otherwise delete it soon. (See also our privacy declaration.) If you wish to receive personal feedback or consultancy from the author, please contact him e.g. via e-mail.
By submitting the information, you give your consent to the potential publication of your inputs on our website according to our rules. (If you later retract your consent, we will delete those inputs.) As your inputs are first reviewed by the author, they may be published with some delay.



These sharing buttons are implemented in a privacy-friendly way!



