Passive Fiber Optics
This is part 2 of a tutorial on passive fiber optics from Dr. Paschotta. The tutorial has the following parts:
1: Guiding light in a glass fiber, 2: Fiber modes, 3: Single-mode fibers, 4: Multimode fibers, 5: Fiber ends, 6: Fiber joints, 7: Propagation losses, 8: Fiber couplers and splitters, 9: Polarization issues, 10: Chromatic dispersion of fibers, 11: Nonlinearities of fibers, 12: Ultrashort pulses and signals in fibers, 13: Accessories and tools
Part 2: Fiber Modes
In general, the intensity profile of light propagating in a fiber changes during propagation. It often even evolves in a rather complicated way. As an example, see what happens if we inject a Gaussian beam, which is tilted by 20° against the beam axis, into a fiber with 20 μm core radius and an NA of 0.3:
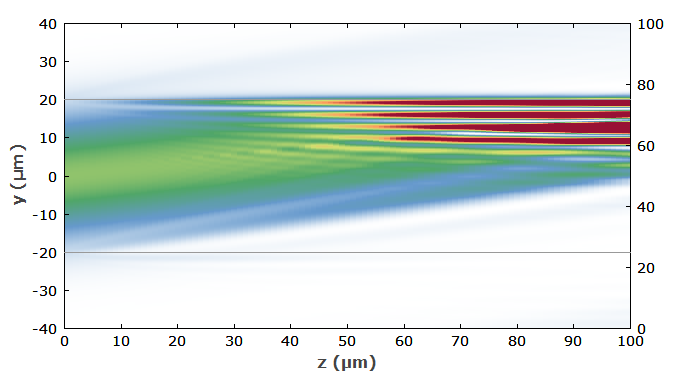
(Note that here we show only intensity profiles, as the larger spatial region shown makes it difficult to show wavefronts.)
One clearly sees the interference effects occurring when the beam reaches the core/cladding interface and is reflected there. At the end, the transverse beam profile looks as shown in Figure 2:
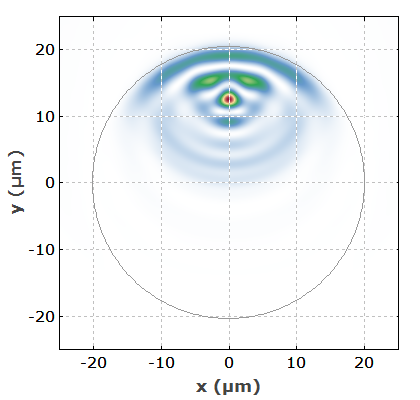
We have seen the intensity profiles generally evolve in complicated ways. However, there are certain amplitude profiles (i.e., distributions of electric field amplitude) where the intensity profile remains unchanged during propagation (assuming a lossless fiber). Such field distributions are called modes of the fiber. The simplest of these, the fundamental mode, also called LP01 mode, looks as follows for the fiber in the current example:
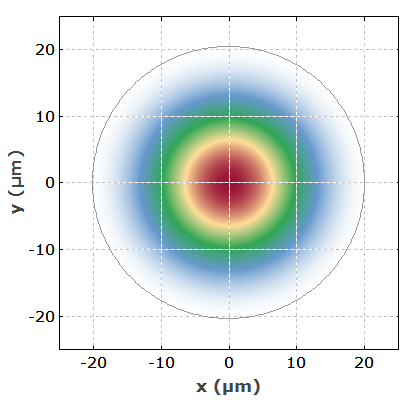
Here is a higher-order mode, the LP37 mode:
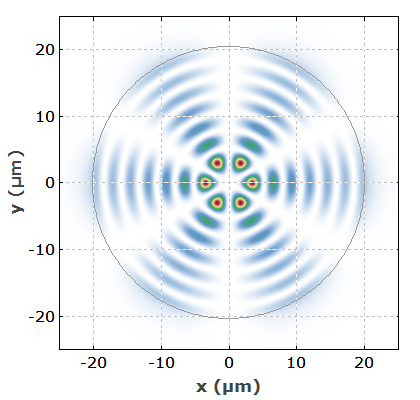
As for the fundamental mode, the natural divergence is exactly canceled by the inhomogeneous index profile.
Note that particularly the higher-order modes can have profiles which significantly extend into the cladding.
The following figure shows the amplitude profiles of all guided modes of the fiber, sorted by their mode indices:
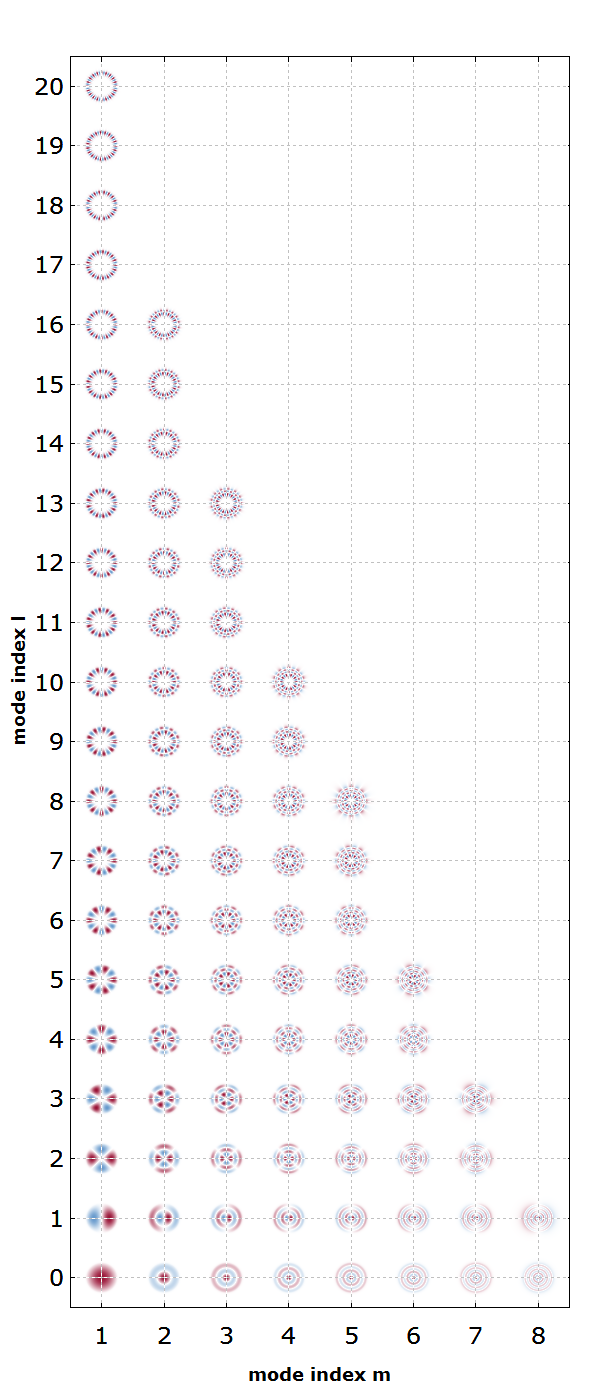
In our example case with 20 μm core radius and NA = 0.3, the fiber has 84 different guided modes at 1.5 μm wavelength (see Figure 5) – when counting different orientations of the modes, there are even 160. (For example, the LP11 mode also exists in a version which is rotated by 90°; these two modes are mutually orthogonal.) All guided modes are essentially restricted to the core region, even though they can somewhat extend into the cladding (but with decreasing intensity for increasing distance from the core).
Figure 6 shows the far field profiles of the fiber modes, as they could be observed in some large distance from the fiber end. They look similar to the near field profiles, but are not simply rescaled versions of them. (You cannot expect that the mode calculated for a certain fiber structure are at the same time modes of free space!) Also note, for example, that although all LP0m modes approximately fill the whole fiber core and thus have similar sizes, the divergence (seen in the far field) gets substantially larger with increasing m.

There are also many non-guided modes, called cladding modes, which can extend throughout the cladding (and the core). As the cladding is typically much larger than the core, and also typically has a higher numerical aperture (due to a large index contrast to a coating around it), the cladding normally has many more modes than the core.
If the refractive index profile has a cylindrical symmetry, we obtain so-called LP modes. These are relatively easily numerically calculated, even for arbitrary radial dependence of the refractive index, and not only for step-index fibers. The RP Fiber Power software, for example, can calculate all the modes of our step-index fiber within a fraction of a second, and would be equally fast for a super-Gaussian radial profile, for example. For not radially symmetric index profiles, much more sophisticated numerical methods are required to calculate all the modes, and that takes much more computation time.
Basic Facts on Modes
We now look at various interesting properties of the modes:
- As mentioned before, the intensity profile of a mode stays constant during propagation in the fiber (see Figure 6), at least if it is lossless. The complex amplitude profile does change, but only in a simple way: the complex phase progresses in proportion to the propagation length: φ = β z with the phase constant β. Note that this phase change applies equally to all transverse positions of the profile. Every mode has its own β value, although mode degeneracies (same β values for different modes) can occur.

- For a lossless fiber, the phase fronts of modes are always plane. The Poynting vector, showing the direction of energy flow, is always parallel to the fiber axis throughout the mode profile.
- If there are propagation losses, e.g. due to a dopant in the fiber core, the modes are somewhat changed. The wavefronts may now get curved, indicating an energy flow in the radial direction. (For example, if we have absorption only in the core, energy must flow from the cladding to the core. In most cases, though, there are only marginal deformations of wavefronts.) The optical power then decreases exponentially along the propagation direction; the propagation constant obtains a real part, which is minus the amplitude absorption coefficient.
- Generally, the number of guided modes increases as the wavelength gets shorter. For long wavelengths, one may have a single guided mode only (→ single-mode fibers), or in fact even no guided mode at all. (A step-index profile always has at least the fundamental mode, but for some photonic crystal fibers this is not the case.)
- The modes form a basis (in the mathematical sense), i.e., a complete orthogonal system, for any guided light. In other words, any complex amplitude distribution which is guided by the fiber can be expressed as a linear superposition of the modes. This mode decomposition can be done for monochromatic light as well as for polychromatic light; in the latter case, one just does the decomposition for each frequency component separately.
Note that a superposition of different modes is generally not a mode itself. Generally, different modes have different β values. Therefore, their phase evolution is different, and the overall intensity profile changes during propagation due to the changing interference conditions. If we have only two modes excited, we get a simple mode beating, where the shape of the intensity profile varies periodically; the period is inversely proportional to the difference in β values. If many modes with different β values are excited, the evolution gets very complicated.
Important Properties of Modes
For a given wavelength, each fiber mode has several important characteristics:
- It has a certain complex amplitude profile, from which one obtains the intensity profile.
- From the intensity profile, one may calculate the effective mode area. This determines how strong nonlinear effects can be.
- The phase constant β tells how fast its overall complex phase changes during propagation. For strongly guided modes, it is well above the phase constant of the cladding. In that case, the intensity profile does not substantially extend into the cladding.
- From the first and second derivative of the β with respect to the optical frequency, one can calculate the group velocity and the group velocity dispersion (GVD).
- For lossy fibers, each mode can have a certain attenuation constant. These values can differ a lot between the modes – sometimes by orders of magnitude. For example, bend losses are often much higher for higher-order modes. They may be calculated with numerical beam propagation or estimated with some analytical techniques. By the way, bending can also shift and distort the mode profile.
- The cut-off wavelength is the wavelength above which the mode ceases to exist. (Under some circumstances, a mode can have a higher and lower cut-off.)
Lower Phase Delays for Higher-order Modes!
Higher-order modes have larger transverse wave vector components than lower-order modes. As a plane-wave component with such a wave vector is tilted against the fiber axis, one may expect that it has an increased path length, so that a higher-order mode will experience a larger phase delay than e.g. the fundamental mode. However, the opposite is true! A larger transverse wave vector component implies a smaller longitudinal component, and that implies a reduced phase delay. So in fact the phase constants (β values) of the highest-order modes are the lowest, and are often close to the refractive index of the cladding times the vacuum wavenumber.
By the way, such modes also exhibit a slow decay of intensity in the cladding, i.e., their intensity distributions extend quite far into the cladding. Another property of these modes is the reduced group velocity (see part 10 on chromatic dispersion). That would actually fit to an increased path length, but the probably surprising findings explained above should be a warning: be careful with quick “explanations” of that vague type.
Calculating the Propagation of Light Based on Modes
Using the calculated set of mode, one can calculate the resulting field profile for an arbitrary input profile after any distance of propagation in the fiber:
- First, calculate the excitation amplitudes of all guided modes via complex overlap integrals of the input amplitude profile with the complex conjugates of all mode amplitude profile.
- Then change the complex amplitudes of all modes according to their β values.
- Construct the final beam profile by adding up all contributions from the modes.
Note that this procedure is not computationally difficult, unless the fiber has a huge number of modes and/or complicated modes in case of an index profile which is not radially symmetric. The amount of computations does not depend on the propagation distance. (For numerical beam propagation, longer distances would generally require more time.)
There are cases where mode coupling calculations are helpful. For example, one may calculate the modes of an “undisturbed” fiber and then calculate mode coupling caused by some additional effect. For example, a periodic index modulation in a fiber Bragg grating can couple counterpropagating or copropagating modes.
Limitations of the Mode Approach
The concept of modes is often very convenient for doing calculations, as shown above. However, it also has its limitations:
- In some cases – for example, for arbitrary index profiles – the modes are very difficult to compute.
- In cases with a huge number of modes, it may also not be convenient to do calculations based on them. Note: if cladding modes need to be taken into account, one would end up with a huge number of modes in the calculation, even if the core has only few guided modes.
- Additional disturbances such as bending already make the calculation of modes much more difficult.
- The concept is also at least more difficult to apply if the mode properties change along the length of a fiber – for example, for tapered fibers.
For such reasons, direct numerical beam propagation is often required instead of the mode concept.
Go to Part 3: Single-mode Fibers or back to the start page.
Questions and Comments from Users
Here you can submit questions and comments. As far as they get accepted by the author, they will appear above this paragraph together with the author’s answer. The author will decide on acceptance based on certain criteria. Essentially, the issue must be of sufficiently broad interest.
Please do not enter personal data here; we would otherwise delete it soon. (See also our privacy declaration.) If you wish to receive personal feedback or consultancy from the author, please contact him e.g. via e-mail.
By submitting the information, you give your consent to the potential publication of your inputs on our website according to our rules. (If you later retract your consent, we will delete those inputs.) As your inputs are first reviewed by the author, they may be published with some delay.



These sharing buttons are implemented in a privacy-friendly way!