RP Fiber Power – Simulation and Design Software
for Fiber Optics, Amplifiers and Fiber Lasers
| Overview | Features | Speed | Model |
| Data | Interface | Demos | Versions |
Example Case: Supercontinuum Generation
Description of the Model
Here, we numerically simulate supercontinuum generation in a germanosilicate fiber. The fiber has been designed such that its zero-dispersion wavelength is not too far from the pump wavelength of 1550 nm, because this allows for better spectral broadening. The mode solver is used to calculate the fiber's effective mode area and chromatic dispersion from the given refractive index profile. The wavelength dependence of refractive indices must be fully taken into account, using Sellmeier equations.
Soliton-shaped pulses with 1 nJ energy and 400 fs duration are injected into the fiber. Supercontinuum generation results from a complicated interplay of fiber nonlinearity and chromatic dispersion. Concerning the nonlinearity, not only the simple Kerr effect is taken into account, but also Raman scattering and self-steepening. The full chromatic dispersion profile (to arbitrarily high orders) is directly taken over from the mode solver. The integrated automatic step size control is useful, since substantially finer steps are required in the last part of the fiber in order to maintain a high accuracy.
Results
Figure 1 shows the wavelength-dependent group velocity dispersion and group index of the fiber, which have been calculated from the refractive index profile. We see that the zero-dispersion wavelength is around 1500 nm, close to the pump wavelength of 1550 nm.
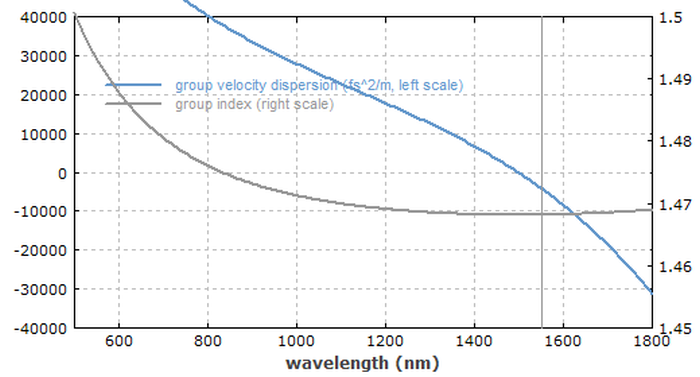
Figure 2 shows the output pulse in the time domain.
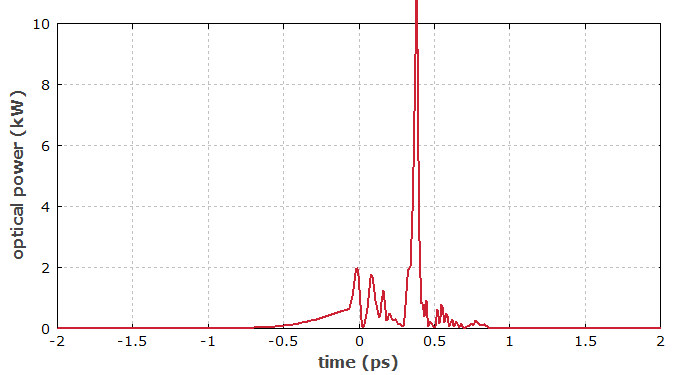
Figure 3 shows the logarithmic spectrum of the output pulse. Substantial spectral broadening is observed.
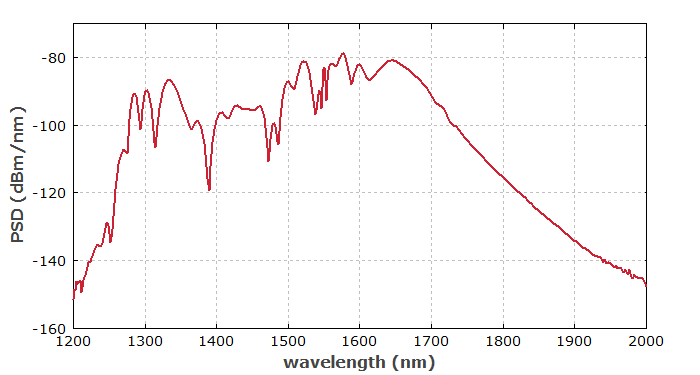
Figure 4 shows how the pulse spectrum evolves within the fiber. Initially, the broadening increases slowly, then more rapidly, and later on there is no more significant further broadening.

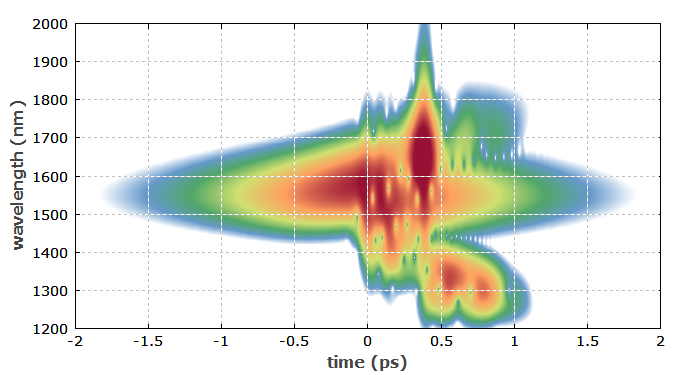
Figure 5 is a spectrogram of the output pulse. The color scale has been made logarithmic, so that weak parts are also visible.