Group Index
Definition: the ratio of the vacuum velocity of light to the group velocity in a medium
Alternative term: group refractive index
German: Gruppenindex
Formula symbol: ng
Units: (dimensionless)
How to cite the article; suggest additional literature
Author: Dr. Rüdiger Paschotta
In analogy with the refractive index, the group index (or group refractive index) ng of a material can be defined as the ratio of the vacuum velocity of light to the group velocity in the medium:
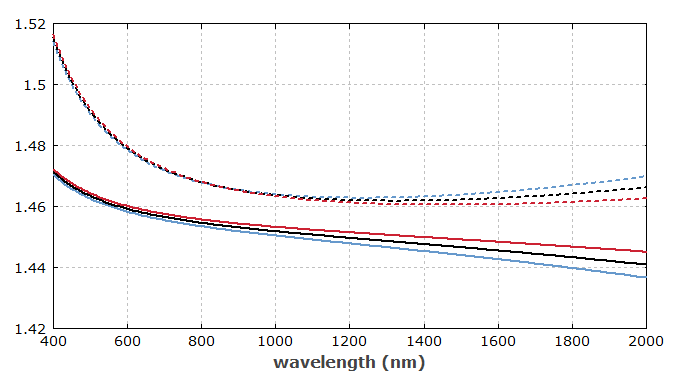
For calculating this, one obviously needs to know not only the refractive index at the wavelength of interest, but also its frequency dependence.
The group index is used, e.g., to calculate time delays for ultrashort pulses propagating in a medium, or the free spectral range of a resonator containing a dispersive medium.
For crystals or glasses, the group index in the visible or near-infrared spectral range is typically larger than the ordinary refractive index, which determines the phase velocity. This implies that the group velocity is often (but not always) lower than the phase velocity.
Note that for optical fibers and other waveguides, one uses the so-called effective refractive index instead of the ordinary refractive index in order to calculate the group velocity, since waveguide dispersion has to be taken into account. Based on that, an effective group index of a fiber could be calculated.
Questions and Comments from Users
Here you can submit questions and comments. As far as they get accepted by the author, they will appear above this paragraph together with the author’s answer. The author will decide on acceptance based on certain criteria. Essentially, the issue must be of sufficiently broad interest.
Please do not enter personal data here; we would otherwise delete it soon. (See also our privacy declaration.) If you wish to receive personal feedback or consultancy from the author, please contact him e.g. via e-mail.
By submitting the information, you give your consent to the potential publication of your inputs on our website according to our rules. (If you later retract your consent, we will delete those inputs.) As your inputs are first reviewed by the author, they may be published with some delay.
See also: group velocity, refractive index
and other articles in the category general optics
 |
If you like this page, please share the link with your friends and colleagues, e.g. via social media: 
These sharing buttons are implemented in a privacy-friendly way! |
2020-04-01
I wonder what is determining the wavelengths of cavity resonances, e.g. in a silicon ring resonator – is it group index or the effective refractive index?
Answer from the author:
In short: a combination of both!
The mode spacing (the frequency spacing of the resonator modes) is determined by the group delay for one resonator round-trip. For a silicon ring resonator, containing a waveguide, the group delay is proportional to the geometrical round-trip length and to the effective group index. Here, “effective” means that we do not simply take a material property, but an effective value calculated for the waveguide structure. Further, “group index” means that we do not simply calculate the effective refractive index, which is relevant only for the phase delay, but the group index, which is relevant for the group delay. That calculation involves the use of frequency derivatives of propagation constants.