Noise in Laser Technology – Part 2: Fluctuations in Pulsed Lasers
Author: Rüdiger Paschotta, RP Photonics Consulting GmbH
(This article appeared in similar form in Optik & Photonik 3, 45 (2009). See also Part 1 – Intensity and Phase Noise and Part 3 – Beam Pointing Fluctuations.)
Abstract: Here, we discuss noise of pulsed lasers, which is in various respects different from that of continuous-wave lasers.
The first part of this series treated noise of the intensity and phase of continuous-wave lasers. Pulsed lasers (e.g. Q-switched lasers) are different in some respects, both concerning the mathematical description and physical features. In some important application areas such as laser material processing, noise properties may be of limited interest, although even in that area there can be impacts e.g. of pulse energy fluctuations on the processing quality. Other pulsed laser systems are used for example for LIDAR, where noise properties can be quite essential.
Beam pointing fluctuations, as can occur in both continuous-wave and pulsed lasers, will be discussed in the next part of this series. Mode-locked lasers are not considered in this article; they do emit pulses, but on a much shorter time scale, and the involved physics are partly quite different.
How to Describe Noise in Pulses
An essential difference between continuous-wave and pulsed lasers is that the intensity, phase etc. of a continuous-wave laser are continuously evolving variables, whereas for pulsed lasers we are dealing with parameters of discrete pulses. Each pulse can be characterized by a set of parameters such as the pulse energy, peak power, duration, mean optical frequency and arrival time, and their fluctuations need to be described statistically.
For single pulses, possibly emitted on demand, the statistical description is relatively simple. For each pulse parameter, there is a distribution function, which may often be approximated with a Gaussian. It is then often sufficient to specify an r.m.s. value of a quantity like the pulse energy under certain conditions (e.g. pulse emission after some minimum pumping time). It may, however, also be relevant to consider correlations between different parameters, as discussed further below.
Frequently, a laser emits pulses periodically with a certain pulse repetition rate frep. The statistics of the fluctuations of pulse parameters can then be described with a power spectral density (PSD) as for a continuous-wave laser, except that the upper noise frequency is limited to frep / 2. Fluctuations faster than that are meaningless. For example, an oscillation of pulse energy with 0.8 frep could not be distinguished from an oscillation with 0.2 frep. On the other hand, the lowest noise frequency on which information can be obtained is again limited by the measurement time: very slow fluctuations are visible only when data are recorded over long times.
Not Trivial: Definition of Pulse Parameters
The definition of some pulse parameters requires careful consideration. For example, the peak power may be taken to be the highest occurring power in a pulse shape, but that depends on a measurement bandwidth. For example, a pulse shape may exhibit substantial mode beating (Fig. 1), which may be seen by a fast photodetector, but averaged out by a slower one. The use of a slow detector may be justified e.g. when the intended application is also not sensitive to fast fluctuations. In some cases, one may even approximate the peak power by the pulse energy divided by the pulse duration.
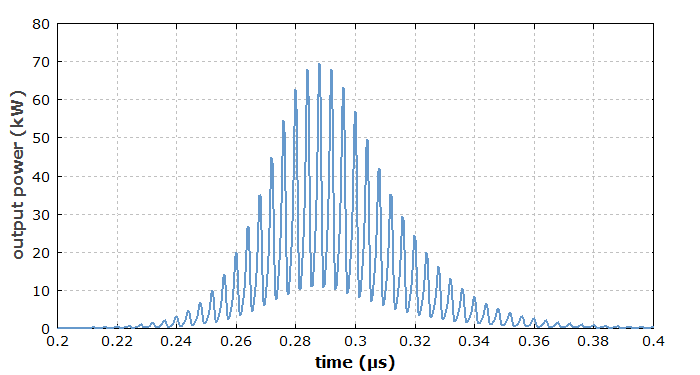
By the way, the pulse duration may be affected by similar issues when mode beating occurs. It should be noted that fluctuations of beam intensity at some point in the profile may be larger than those of the power, as the spatial beam shape may also fluctuate. This may happen in lasers emitting on multiple transverse modes – particularly when the number of involved modes is small.
Timing errors can also be defined in different ways. First of all, what is the temporal position of a pulse? The start of a pulse can not be reliably determined, and the peak (position with highest power) is also problematic: it can depend on the detection bandwidth and can even be ambiguous. The most common definition of temporal pulse position, avoiding such problems, is based on the “center of mass” of the power distribution. Its fluctuations will often be substantially weaker than those of the peak position. The next question is how to define the timing error. For an actively Q-switched laser, one may consider the fluctuations of the delay between a trigger signal and the pulse position. In other cases, one may refer to a regular timing grid with the same average repetition rate.
Relevant Physical Phenomena in Pulsed Lasers
In pulsed lasers, a wide range of physical phenomena are responsible for fluctuations. In Q-switched lasers, for example, the pulse build-up normally starts with spontaneous emission, and its randomness causes macroscopic fluctuations of pulse parameters – particularly of the pulse timing and (for multimode lasers) the distribution of pulse energy over different resonator modes. Further influences come from thermal changes, mechanical vibrations, pump noise, etc.

A fiber amplifier system, generating pulses with similar parameters but in a very different way (Fig. 2), can have entirely different noise properties. For example, the pulse timing may be much more precise, and higher-order modes are absent if single-mode fiber is used. On the other hand, the linewidth may be by orders of magnitude larger than that of a single-mode Q-switched bulk laser, and nonlinear effects in the power amplifier may generate spurious Raman-shifted wavelength components.
Some pulsed solid-state lasers are not Q-switched, but free-running with a pulsed pump source. Particularly high pulse energies can be associated with strong heating effects during a pulse, which can have many consequences, such as changes of mode frequencies, the spectral profile of the gain, thermal lensing, etc. Therefore, such lasers are hardly candidates for particularly low-noise operation.
The best noise properties are often achieved in lasers with tight mode control. For example, a compact Q-switched Nd:YAG laser may have a resonator with good suppression of higher-order transverse modes and a high frequency selectivity, enforcing stable operation on a single resonator mode (single-frequency operation). This fully eliminates a range of noise phenomena associated with mode competition, mode beating etc. If the setup is also mechanically stable, and the laser is pumped with a low-noise laser diode, the noise performance may be excellent particularly in terms of pulse energy, duration and linewidth. Further improvements may result from active stabilization of the optical frequency, possibly combined with some prelasing and/or injection locking technique. Here, the randomness in the pulse initiation is reduced by providing a well-defined seed signal.
Noise Influences in Pulse Amplification
The limited pulse energy from compact single-frequency lasers sometimes enforces the use of an amplifier system. Unfortunately, amplification can introduce various kinds of noise. As long as an amplifier is only weakly saturated, the pulse energy fluctuations may be increased strongly, essentially because tiny fluctuations of stored energy translate into substantial fluctuations of amplifier gain. That problem becomes smaller in strongly saturated amplifiers; this situation is common in power amplifier stages and particularly for fiber amplifiers. Here, the output energy is essentially limited by the extractable energy, and not by the unsaturated laser gain.
A saturated amplifier may still suffer from nonlinear effects – particularly when it is a fiber amplifier. Stimulated Raman scattering (SRS) can shift a fluctuating amount of energy to longer wavelengths, whereas stimulated Brillouin scattering (SBS) leads to back-reflected energy, and the Kerr nonlinearity may redistribute power between different modes or even create new frequency components via four-wave mixing, also involving quantum noise. It may even be necessary to use a seed laser with a large linewidth in order to avoid problems with SBS.
Even a perfect amplifier can not avoid the problem that a low-power seed laser may have a fundamentally limited noise performance. For example, a low-power laser diode has a much larger linewidth than an optimized compact Q-switched bulk laser, and that problem may only become worse during amplification. For excellent noise properties at high power levels, one may have to resort to injection seeding of carefully stabilized high-power lasers.
Correlated Fluctuations
As mentioned above, correlations can also be of interest, and they occur frequently. In Q-switched lasers, for example, the fluctuations of pulse energy, duration and pulse timing are normally correlated: the highest-energy pulses usually come sooner and are shorter. This is because an above-average stored energy in the gain medium before the pulse causes a higher pulse energy, but also a larger laser gain and thus faster pulse build-up. As a result, the relative fluctuations of peak power are larger than those of the pulse energy.
Conclusions
Compared to continuous-wave lasers, pulsed lasers tend to have more complex noise properties. They exhibit many more possible types of noise, as there are plenty of fluctuating parameters, compared to just two (intensity and phase) for a single-frequency continuous-wave laser. Also, a lot of additional physical phenomena in pulsed lasers can be relevant for the noise performance.
Depending on the application, different types of noise can be relevant, and different types of laser systems can be very different in such respects. We have seen, for example, that a fiber amplifier system may deliver much more precise pulse timing, while not being competitive in terms of a small linewidth in energetic pulses. Therefore, it can be crucial to well understand both the detailed requirements of an application and the strengths and weaknesses of different kinds of laser systems.
Questions and Comments from Users
Here you can submit questions and comments. As far as they get accepted by the author, they will appear above this paragraph together with the author’s answer. The author will decide on acceptance based on certain criteria. Essentially, the issue must be of sufficiently broad interest.
Please do not enter personal data here; we would otherwise delete it soon. (See also our privacy declaration.) If you wish to receive personal feedback or consultancy from the author, please contact him e.g. via e-mail.
By submitting the information, you give your consent to the potential publication of your inputs on our website according to our rules. (If you later retract your consent, we will delete those inputs.) As your inputs are first reviewed by the author, they may be published with some delay.
Bibliography
| [1] | RP Photonics Encyclopedia: articles on laser noise, intensity noise, phase noise and others |
| [2] | R. Paschotta, H. R. Telle, and U. Keller, Noise of Solid State Lasers, in Solid-State Lasers and Applications (ed. A. Sennaroglu), CRC Press, Boca Raton, FL (2007), Chapter 12, pp. 473–510 |
| [3] | Part 1 – Intensity and Phase Noise |
| [4] | Part 3 – Beam Pointing Fluctuations |
See also: pulsed lasers, Q-switched lasers, pulse energy, peak power, pulse duration, timing jitter
