Self-focusing

As the decrease in the beam radius further increases the strength of the Kerr lens, there may be total collapse of the beam: as the beam radius is reduced, the optical intensities become even higher, further increasing the self-focusing effect. This mechanism can lead to very high optical intensities which can easily destroy the optical medium (optical damage). Such a run-away effect can occur when the optical power is above the critical power

where n₂ is the nonlinear index, λ the vacuum wavelength, and n the refractive index. This holds for linearly polarized light. For circularly polarized light, the self-focusing behavior can be substantially different [7].

Remarkably, the critical power does not depend on the original beam area. (A larger beam generates a weaker Kerr lens, but it is also more sensitive to lensing.) Only, an initially larger beam will require a longer propagation distance (for a given optical power) until it collapses. For silica (as used e.g. in silica fibers), the self-focusing limit in terms of peak power is of the order of 4 MW in the 1-μm wavelength region.

Calculator for Self-focussing

Wavelength:
Refractive index:
Nonlinear index:
Critical power:				calc

Enter input values with units, where appropriate. After you have modified some inputs, click the "calc" button to recalculate the output.

Note that the value used for the nonlinear index depends on the pulse duration. For shorter pulses, a somewhat lower value must be used, as there is not sufficient time for the effect of electrostriction and possibly for the delayed nonlinear response (→ Raman scattering).

In an optical fiber, the built-in waveguide forms guided modes. Self-focusing then reduces the effective mode area (see Figure 1). At the critical power (which is the same as for bulk material), the mode area approaches zero.

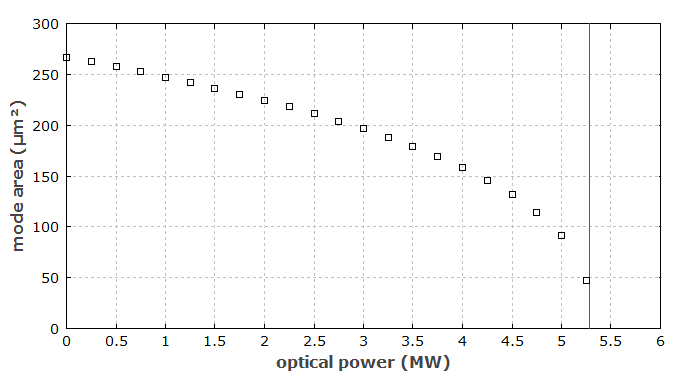
A beam in a homogeneous medium (i.e., with no waveguide) with a power exactly at the self-focusing limit could theoretically exhibit self-trapping [1], where the beam profile stays constant over a longer distance, because divergence is exactly compensated by the nonlinear focusing effect. That state, however, is unstable; small deviations from that state would grow rapidly.

Higher-order modes also become unstable as a consequence of the nonlinear interaction. Figure 2 shows an example, where a power of 4 MW has been injected into the LP₁₁ mode of a fiber. After about 10 mm of propagation distance, the light is transferred into a mixture of LP₀₁ and LP₁₁.

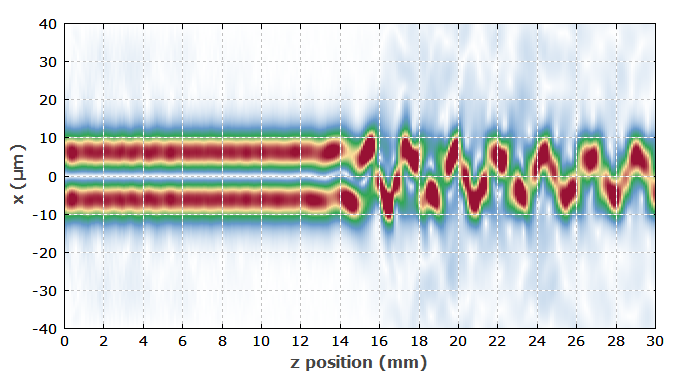
For optical powers far above the self-focusing limit, filamentation can occur, where the beam breaks up into several beams with smaller powers. The resulting beam pattern can be random, but in some cases it has a fairly regular structure.

Different dynamics (transient self-focusing [3]) occur for ultrashort pulses when the pulse duration is not much longer than the characteristic time scale of the nonlinearity [3].

The reduction in beam radius for high intensities (for peak powers below the self-focusing limit) can be used for Kerr lens mode locking of a laser, when it leads to a better overlap of laser and pump beam, or to reduced losses at some aperture. In both cases, it generates a kind of artificial saturable absorber.

Another application of self-focusing is the measurement of the magnitude of the Kerr nonlinearity (→ z-scan measurements).

Note that cascaded χ⁽²⁾ nonlinearities (essentially, frequency doubling with subsequent backconversion) can also lead to an effective χ⁽³⁾ nonlinearity, resulting either in self-focusing or (for negative effective χ⁽³⁾) self-defocusing.

Your question or comment:

Spam check:

  (Please enter the sum of thirteen and three in the form of digits!)

By submitting the information, you give your consent to the potential publication of your inputs on our website according to our rules. (If you later retract your consent, we will delete those inputs.) As your inputs are first reviewed by the author, they may be published with some delay.

[1]	R. Y. Chiao, E. Garmire, and C. H. Townes, “Self-trapping of optical beams”, Phys. Rev. Lett. 13 (15), 479 (1964), doi:10.1103/PhysRevLett.13.479
[2]	P. L. Kelley, “Self-focusing of optical beams”, Phys. Rev. Lett. 15 (26), 1005 (1965), doi:10.1103/PhysRevLett.15.1005 (see also the erratum in Phys. Rev. Lett. 16, 384 (1966))
[3]	Y. R. Shen, “Self-focusing: experimental”, Prog. Quantum Electron. 4, 1 (1975), doi:10.1016/0079-6727(75)90002-6
[4]	J. H. Marburger, “Self-focusing: theory”, Prog. Quantum Electron. 4, 35 (1975), doi:10.1016/0079-6727(75)90003-8
[5]	G. Cerullo et al., “Space-time coupling and collapse threshold for femtosecond pulses in dispersive nonlinear media”, Opt. Lett. 21 (1), 65 (1996), doi:10.1364/OL.21.000065
[6]	G. Fibich and A. L. Gaeta, “Critical power for self-focusing in bulk media and in hollow waveguides”, Opt. Lett. 25 (5), 335 (2000), doi:10.1364/OL.25.000335
[7]	G. Fibich and B. Ilan, “Self-focusing of circularly polarized beams”, Phys. Rev. E 67 (3), 036622 (2003), doi:10.1103/PhysRevE.67.036622
[8]	R. L. Farrow et al., “Peak-power limits on fiber amplifiers imposed by self-focusing”, Opt. Lett. 31 (23), 3423 (2006), doi:10.1364/OL.31.003423
[9]	L. Dong, “Approximate treatment of the nonlinear waveguide equation in the regime of nonlinear self-focus”, J. Lightwave Technol. 26 (20), 3476 (2008), doi:10.1109/JLT.2008.925685
[10]	A. V. Smith et al., “Optical damage limits to pulse energy from fibers”, J. Sel. Top. Quantum Electron. 15 (1), 153 (2009), doi:10.1109/JSTQE.2008.2010331
[11]	D. E. Laban et al., “Self-focusing in air with phase-stabilized few-cycle light pulses”, Opt. Lett. 35 (10), 1653 (2010), doi:10.1364/OL.35.001653
[12]	P. Whalen et al., “Self-focusing collapse distance in ultrashort pulses and measurement of nonlinear index”, Opt. Lett. 36 (13), 2542 (2011), doi:10.1364/OL.36.002542
[13]	S. A. Kozlov et al., “Suppression of self-focusing for few-cycle pulses”, J. Opt. Soc. Am. B 36 (10), G68, doi:10.1364/JOSAB.36.000G68
[14]	R. Paschotta, tutorial on "Passive Fiber Optics", Part 11: Nonlinearities of Fibers

(Suggest additional literature!)