Coherent States
Definition: a special kind of pure quantum-mechanical states of light
Alternative term: Glauber states
German: kohärente Zustände
Categories: quantum optics, fluctuations and noise
How to cite the article; suggest additional literature
Author: Dr. Rüdiger Paschotta

The term coherent state (also called Glauber state) has been introduced by Roy J. Glauber in 1963 [1] (Glauber received the Nobel Prize in physics in 2005 for work related to coherent states). It is not strongly related to the classical term coherence, and refers to a special kind of pure quantum-mechanical state of the light field corresponding to a single resonator mode. It is defined by
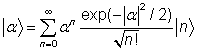
i.e., as a coherent superposition of photon number states (Fock states). The complex parameter α determines the average photon number (which is its squared modulus) and the phase of the coherent state.
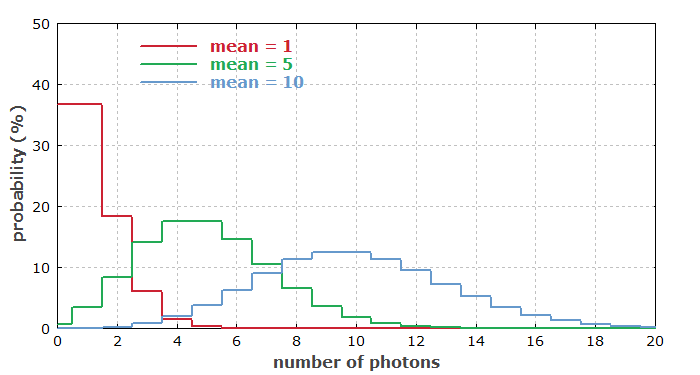
From the above equation one easily finds that the probability of finding n photons in a coherent state is given by
where <n> = |α|2 is the mean photon number. This shows that the coherent state exhibits Poissonian photon statistics. The figure below shows the distribution of probabilities for different mean photon numbers. For large mean photon numbers (e.g., well above 10), the distribution can be approximated by a Gaussian function, where the variance equals the mean photon number.
In some respects, the coherent state has properties which are relatively close to a classical state of the light field. For example, it resembles a classical oscillation of the light field, apart from some superimposed quantum noise, which is relatively weak for large average photon numbers. The quantum noise of the quadrature components of a Glauber state is equal. A nonlinear interaction can transform the circular uncertainty area into a deformed area with lower noise in one quadrature component; such states are called squeezed states of light. When such light fields experience linear losses, they are again pulled toward a coherent state.
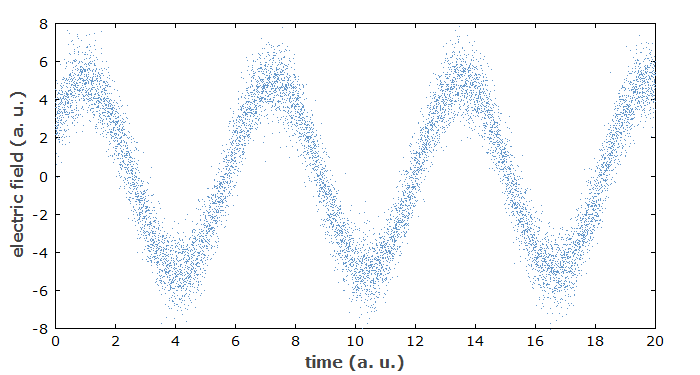
The output of a single-frequency laser well above threshold can approach a coherent state, if the long-term phase drift (related to the Schawlow–Townes linewidth) is disregarded. This is valid for high noise frequencies.
A special case of a Glauber state is that with α = 0. This is the vacuum state, having a photon number of zero, but still exhibiting quantum fluctuations of the electric and magnetic field, sometimes called vacuum noise.
Questions and Comments from Users
Here you can submit questions and comments. As far as they get accepted by the author, they will appear above this paragraph together with the author’s answer. The author will decide on acceptance based on certain criteria. Essentially, the issue must be of sufficiently broad interest.
Please do not enter personal data here; we would otherwise delete it soon. (See also our privacy declaration.) If you wish to receive personal feedback or consultancy from the author, please contact him e.g. via e-mail.
By submitting the information, you give your consent to the potential publication of your inputs on our website according to our rules. (If you later retract your consent, we will delete those inputs.) As your inputs are first reviewed by the author, they may be published with some delay.
Bibliography
| [1] | R. J. Glauber, “Coherent and incoherent states of the radiation field”, Phys. Rev. 131 (6), 2766 (1963), doi:10.1103/PhysRev.131.2766 |
| [2] | Wikipedia article on coherent states |
See also: quantum optics, quantum noise, vacuum noise, squeezed states of light, Schawlow–Townes linewidth
and other articles in the categories quantum optics, fluctuations and noise
 |



If you like this page, please share the link with your friends and colleagues, e.g. via social media:
These sharing buttons are implemented in a privacy-friendly way!