Principal Points and Principal Planes
Definition: planes from which rays appear to be deflected in an optical system according to paraxial optics, and the intersections of those planes with the optical axis
German: Hauptebenen
Categories: general optics, vision, displays and imaging
How to cite the article; suggest additional literature
Author: Dr. Rüdiger Paschotta
The principal points (not principle points!) and principal planes of an optical system play a fundamental role in Gaussian optics; the principal points of an optical element or a combination of elements are part of its cardinal points. In the following, it is first explained how they are defined.
Within paraxial optics, one can use the ABCD matrix algorithm for calculating the change of beam offset and direction caused by some optical element or a complete optical system consisting of several elements.
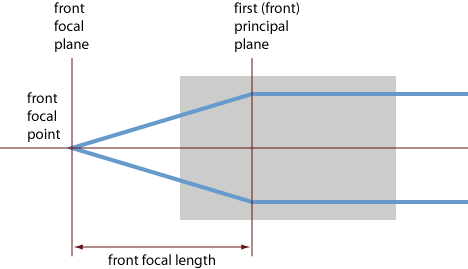
We first consider a situation where the output rays are parallel to the optical axis. Ignoring the actual beam path within the optical system, one can now extrapolate the ingoing and outgoing rays such that they meet in a plane, and that plane is called the first principal plane H, also called front principal plane (see Figure 1).
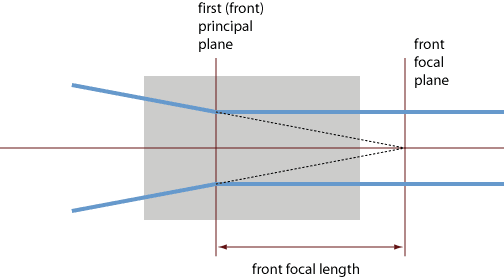
For a defocusing system (Figure 2), the first focal plane can be located on the output side; it contains a virtual focal point.
Similarly, we can consider a situation where the input rays are parallel to the optical axis. By again extrapolating the ingoing and outgoing rays, one finds the second principal plane H', also called the back principal plane (see Figure 3).
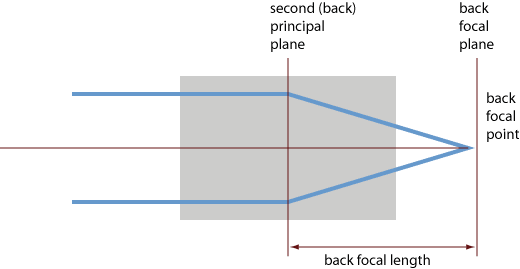
In each case, one obtains a focal length as the distance between a principal plane and the corresponding focal plane. If the refractive index of the input and output medium is the same, the two values of the focal length are identical – apart from possible sign differences, depending on the used sign convention.
The principal points are the points where the principal planes intersect with the optical axis. Together with focal points and nodal points, they belong to the cardinal points.
Note that although the figures in this article have been made with substantial transverse offsets and angles of the rays, the principal planes are defined based on paraxial optics, i.e., for the limiting case of small transverse offset and angles. Actual paths of rays with more extreme coordinates can somewhat deviate.
The locations of the left and right edges of the optical system (e.g. positions of outer lens surfaces, optical windows etc.) or its housing are not relevant for those definitions. Unfortunately, the positions of the principal planes e.g. of a photographic objective are normally not made known to the user, so that it would not be possible to locate the focal point without optical measurements.
The positions of the principal planes can be calculated from the ABCD matrix of the system. Conversely, one can calculate that matrix from the positions of the principal planes and the focal planes.
Example Cases
Some relations for lenses:
- For an infinitely thin lens (a purely theoretical construction), both principal planes are at the lens position.
- For a thick biconvex lens, they do not coincide and lie somewhere within the lens.
- For a plano-convex lens, one principal plane touches the curved surface, and the other one lies within the lens.
- For a meniscus lens, the principal planes lie outside the lens.
Questions and Comments from Users
Here you can submit questions and comments. As far as they get accepted by the author, they will appear above this paragraph together with the author’s answer. The author will decide on acceptance based on certain criteria. Essentially, the issue must be of sufficiently broad interest.
Please do not enter personal data here; we would otherwise delete it soon. (See also our privacy declaration.) If you wish to receive personal feedback or consultancy from the author, please contact him e.g. via e-mail.
By submitting the information, you give your consent to the potential publication of your inputs on our website according to our rules. (If you later retract your consent, we will delete those inputs.) As your inputs are first reviewed by the author, they may be published with some delay.
See also: geometrical optics, ABCD matrix, paraxial approximation, focal length
and other articles in the categories general optics, vision, displays and imaging
 |



If you like this page, please share the link with your friends and colleagues, e.g. via social media:
These sharing buttons are implemented in a privacy-friendly way!