Chromatic Aberrations

A frequently encountered phenomenon is that the axial (longitudinal) position of a focus or a sharp image depends on the optical wavelength; such distortions are called axial chromatic aberrations. They are related to the wavelength dependence of the focal length (and thus the dioptric power) of an optical system. In photography, their impact can be reduced by working with an aperture of smaller size, i.e., a higher f-stop number.

As an example case, we consider collimated laser beams with an initial beam radius of 1 mm, hitting a biconvex lens made of BK7 glass. Both lens surfaces are assumed to have a radius of curvature of 103 mm. Due to the moderate chromatic dispersion of that crown glass, the focus positions vary substantially (see Figure 1) – the deviations are of the order of the Rayleigh length in this case.

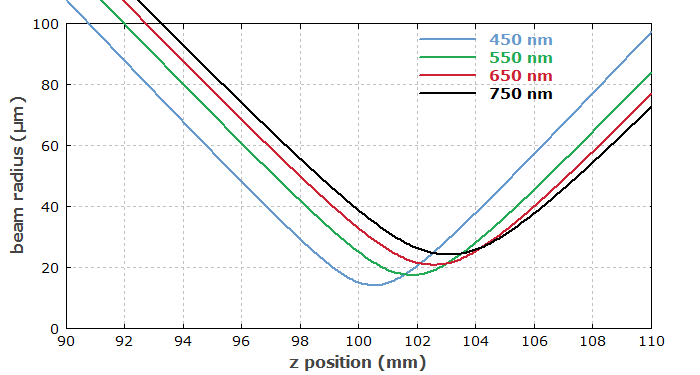
Note that the also observed variation of beam radius in the focus is essentially not caused by the material dispersion, but by diffraction.

Figure 2 shows the same for an achromatic doublet made from BK7 crown glass and SF2 flint glass with a numerically optimized design. The wavelength dependence of the beam radius is essentially not caused by the material dispersion, but by diffraction.

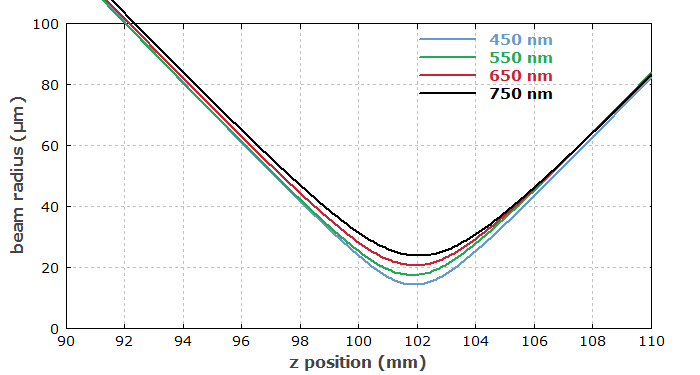
There are also so-called transverse aberrations, denoting the wavelength dependence of transverse positions e.g. of image details. They can arise from the wavelength dependence of the image magnification or of distortions. Such effects do not occur at the center of an image and generally increase with the distance from that center. (In contrast, axial aberrations affect the whole image.) An increased f-stop number does not reduce transverse aberrations.

Traditionally, chromatic aberrations in the visible spectral region are quantified based on measurements at three wavelengths:

• λ_F = 486.1 nm (blue Fraunhofer F line from hydrogen)
• λ_D = 589.2 nm (orange Fraunhofer D line from sodium)
• λ_C = 656.3 nm (red Fraunhofer C line from hydrogen)

These wavelengths span much of the visible spectral region, and the middle one (the D line) lies in the region of maximum sensitivity of the human eye. The refractive indices of the material at these wavelengths are called n_F, n_D and n_C, respectively. In some cases, somewhat different wavelength values corresponding to other Fraunhofer lines are used, e.g., 480.0 nm (F' line), 587.6 nm (d line) and 643.9 nm (C' line); note that light with some wavelengths is easier to produce in gas discharge lamps than other wavelengths.

An important parameter in the context of chromatic aberrations is the Abbe number, which is defined as

Obviously, the Abbe number becomes small (rather than large) for materials with a strong wavelength dependence of the refractive index. Glasses with a relatively low Abbe number of less than 50 (i.e., with relatively strong dispersion) are called flint glasses, whereas glasses with a higher Abbe number are crown glasses. Typically, flint glasses have relatively high refractive indices, whereas crown glasses exhibit lower values. One might think that one should avoid the use of flint classes in order to obtain low chromatic aberrations; in reality, however, proper combinations of crown and flint glasses are often used for realizing achromatic optics.

Abbe numbers appear in various formulas for the calculation of optical aberrations, e.g. of an axial mismatch of focus or image positions at certain wavelengths. Such equations can then also be used to calculate parameter combinations for which certain chromatic aberrations vanish (→ achromatic optics). Note that particularly for various kinds of transverse chromatic aberrations, related to the wavelength dependence of various types of imaging distortions, the required equations can become relatively complicated. Therefore, the optimization of optical systems often requires sophisticated numerical optimization strategies.

Of course, calculations based on only three optical wavelengths cannot provide complete information on chromatic aberrations over the whole visible spectral region or even on other spectral regions; the optimization of optical systems often requires one to take into account refractive index data for additional wavelength values.

Even achromatic optics typically achieve a perfect suppression of axial and/or transverse chromatic aberrations only for two or three different wavelengths, while exhibiting more less pronounced distortions at other wavelengths. Note also that a comprehensive optimization of an optical system requires a compromise between different qualities, of which the strength of chromatic aberrations is only one.

Your question or comment:

Spam check:

  (Please enter the sum of thirteen and three in the form of digits!)

By submitting the information, you give your consent to the potential publication of your inputs on our website according to our rules. (If you later retract your consent, we will delete those inputs.) As your inputs are first reviewed by the author, they may be published with some delay.