Conjugate Planes
Definition: pairs of planes where an optical system images one into the other and vice versa
German: konjugigerte Ebenen
Category: vision, displays and imaging
How to cite the article; suggest additional literature
Author: Dr. Rüdiger Paschotta
An optical imaging system often works such that all points in a certain plane are imaged into points of another plane. At least within geometrical optics, there is then a one-to-one correspondence between points in the two planes: One point in one of the planes is mapped onto a certain point on the other plane, and vice versa, as shown in Figure 1. The two planes are then called conjugate to each other.
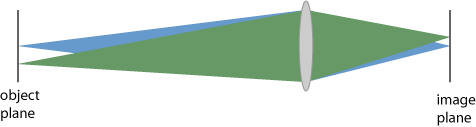
Conjugate planes usually exist only within the paraxial approximation. For larger ray angles against the optical axis, the image points corresponding to points in one plane are often found to lie on a curved surface. This phenomenon is related to image distortion, as explained in the article on optical aberrations.
Just like conjugate planes, there are also conjugate points – one point in a plane is conjugate to another point in the conjugate plane.
Conjugate Planes in a Microscope
Conjugate planes do not only come in pairs; there can be subsequent imaging stages e.g. in a microscope, creating multiple planes which are conjugate to each other. One may also distinguish different sets of conjugate plane in a microscope:
- An image-forming plane set has planes at the specimen, an intermediate image plane near the eyepiece, and the retina of the observing eye, and normally also a plane in the illumination beam path.
- There is also an illumination plane set with the lamp filament (typically of a halogen lamp), condenser diaphragm, objective back focal plane and eye iris.
Those two plane sets are usually separated such that the structure of the lamp filament is not significantly affecting the image of the specimen (principle of Köhler illumination): the plane of the lamp filament must be far from being conjugate to the specimen plane.
Conjugate Planes at Infinity
In a generalization of the concept, one or both of the planes can lie at infinity (i.e., at infinite distance from the imaging system). This can be the case for a telescope in its basic afocal configuration, where objects in an infinite distance are mapped to an image which is also at infinite distance. In other words, parallel incoming light rays are transformed into parallel rays at the output, just with some linear and angular magnification. In a modified configuration, there can be an image plane at a finite distance on one side, where an image sensor can be placed, for example. Further, a slight increase of the distance between objective and ocular lens can also bring the object plane to a finite distance.
Questions and Comments from Users
Here you can submit questions and comments. As far as they get accepted by the author, they will appear above this paragraph together with the author’s answer. The author will decide on acceptance based on certain criteria. Essentially, the issue must be of sufficiently broad interest.
Please do not enter personal data here; we would otherwise delete it soon. (See also our privacy declaration.) If you wish to receive personal feedback or consultancy from the author, please contact him e.g. via e-mail.
By submitting the information, you give your consent to the potential publication of your inputs on our website according to our rules. (If you later retract your consent, we will delete those inputs.) As your inputs are first reviewed by the author, they may be published with some delay.
See also: image planes, imaging with a lens, geometrical optics, paraxial approximation
and other articles in the category vision, displays and imaging
 |




If you like this page, please share the link with your friends and colleagues, e.g. via social media:
These sharing buttons are implemented in a privacy-friendly way!